Need help? We're here to assist you!
Thank You for Enquiry, we will contact you soon!
Close
The Class 8 is an important year in a student’s life and Maths is one of the subjects that require dedication, hard work, and practice. It’s a subject where you can score well if you are well-versed with the concepts, remember the important formulas and solving methods, and have done an ample amount of practice. Worry not! Home Revise is here to make your Class 8 journey even easier. It’s essential for students to have the right study material and notes to prepare for their board examinations, and through Home Revise, you can cover all the fundamental topics in the subject and the complete NCERT Class 8 Maths Book syllabus.

Exercise 7.1 Page: 114
1. Which of the following numbers are not perfect cubes?
(i) 216
Solution:
By resolving 216 into a prime factor,
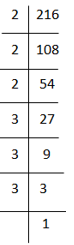
216 = 2×2×2×3×3×3
By grouping the factors in triplets of equal factors, 216 = (2×2×2)×(3×3×3)
Here, 216 can be grouped into triplets of equal factors,
∴ 216 = (2×3) = 6
Hence, 216 is the cube of 6.
(ii) 128
Solution:
By resolving 128 into a prime factor,
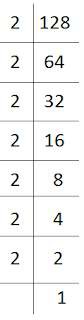
128 = 2×2×2×2×2×2×2
By grouping the factors in triplets of equal factors, 128 = (2×2×2)×(2×2×2)×2
Here, 128 cannot be grouped into triplets of equal factors, and we are left with one factor: 2.
∴ 128 is not a perfect cube.
(iii) 1000
Solution:
By resolving 1000 into prime factor,
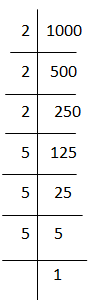
1000 = 2×2×2×5×5×5
By grouping the factors in triplets of equal factors, 1000 = (2×2×2)×(5×5×5)
Here, 1000 can be grouped into triplets of equal factors.
∴ 1000 = (2×5) = 10
Hence, 1000 is the cube of 10.
(iv) 100
Solution:
By resolving 100 into a prime factor,
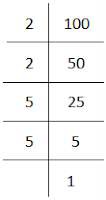
100 = 2×2×5×5
Here, 100 cannot be grouped into triplets of equal factors.
∴ 100 is not a perfect cube.
(v) 46656
Solution:
By resolving 46656 into prime factor,
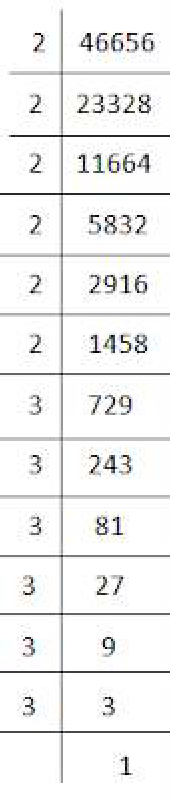
46656 = 2×2×2×2×2×2×3×3×3×3×3×3
By grouping the factors in triplets of equal factors, 46656 = (2×2×2)×(2×2×2)×(3×3×3)×(3×3×3)
Here, 46656 can be grouped into triplets of equal factors,
∴ 46656 = (2×2×3×3) = 36
Hence, 46656 is the cube of 36.
2. Find the smallest number by which each of the following numbers must be multiplied to obtain a perfect cube.
(i) 243
Solution:
By resolving 243 into a prime factor,
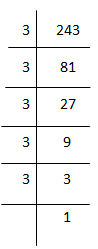
243 = 3×3×3×3×3
By grouping the factors in triplets of equal factors, 243 = (3×3×3)×3×3
Here, 3 cannot be grouped into triplets of equal factors.
∴ We will multiply 243 by 3 to get the perfect cube.
(ii) 256
Solution:
By resolving 256 into a prime factor,
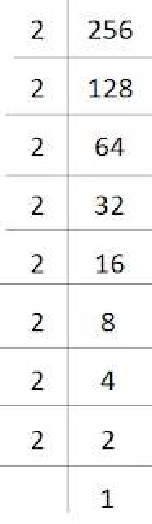
256 = 2×2×2×2×2×2×2×2
By grouping the factors in triplets of equal factors, 256 = (2×2×2)×(2×2×2)×2×2
Here, 2 cannot be grouped into triplets of equal factors.
∴ We will multiply 256 by 2 to get the perfect cube.
(iii) 72
Solution:
By resolving 72 into a prime factor,
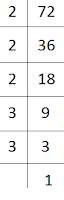
72 = 2×2×2×3×3
By grouping the factors in triplets of equal factors, 72 = (2×2×2)×3×3
Here, 3 cannot be grouped into triplets of equal factors.
∴ We will multiply 72 by 3 to get the perfect cube.
(iv) 675
Solution:
By resolving 675 into a prime factor,
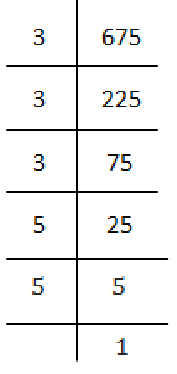
675 = 3×3×3×5×5
By grouping the factors in triplets of equal factors, 675 = (3×3×3)×5×5
Here, 5 cannot be grouped into triplets of equal factors.
∴ We will multiply 675 by 5 to get the perfect cube.
(v) 100
Solution:
By resolving 100 into a prime factor,
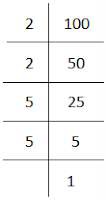
100 = 2×2×5×5
Here, 2 and 5 cannot be grouped into triplets of equal factors.
∴ We will multiply 100 by (2×5) 10 to get the perfect cube.
3. Find the smallest number by which each of the following numbers must be divided to obtain a perfect cube.
(i) 81
Solution:
By resolving 81 into a prime factor,
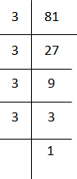
81 = 3×3×3×3
By grouping the factors in triplets of equal factors, 81 = (3×3×3)×3
Here, 3 cannot be grouped into triplets of equal factors.
∴ We will divide 81 by 3 to get the perfect cube.
(ii) 128
Solution:
By resolving 128 into a prime factor,

128 = 2×2×2×2×2×2×2
By grouping the factors in triplets of equal factors, 128 = (2×2×2)×(2×2×2)×2
Here, 2 cannot be grouped into triplets of equal factors.
∴ We will divide 128 by 2 to get the perfect cube.
(iii) 135
Solution:
By resolving 135 into prime factor,
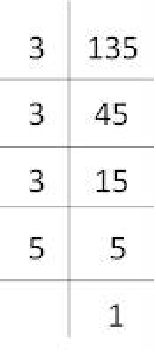
135 = 3×3×3×5
By grouping the factors in triplets of equal factors, 135 = (3×3×3)×5
Here, 5 cannot be grouped into triplets of equal factors.
∴ We will divide 135 by 5 to get the perfect cube.
(iv) 192
Solution:
By resolving 192 into a prime factor,
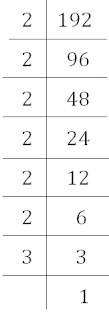
192 = 2×2×2×2×2×2×3
By grouping the factors in triplets of equal factors, 192 = (2×2×2)×(2×2×2)×3
Here, 3 cannot be grouped into triplets of equal factors.
∴ We will divide 192 by 3 to get the perfect cube.
(v) 704
Solution:
By resolving 704 into a prime factor,

704 = 2×2×2×2×2×2×11
By grouping the factors in triplets of equal factors, 704 = (2×2×2)×(2×2×2)×11
Here, 11 cannot be grouped into triplets of equal factors.
∴ We will divide 704 by 11 to get the perfect cube.
4. Parikshit makes a cuboid of plasticine with sides 5 cm, 2 cm, and 5 cm. How many such cuboids will he need to form a cube?
Solution:
Given the sides of the cube are 5 cm, 2 cm and 5 cm.
∴ Volume of cube = 5×2×5 = 50
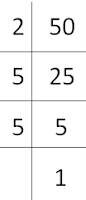
50 = 2×5×5
Here, 2 , 5 and 5 cannot be grouped into triplets of equal factors.
∴ We will multiply 50 by (2×2×5) 20 to get the perfect cube. Hence, 20 cuboids are needed.
1. Find the cube root of each of the following numbers by the prime factorisation method.
(i) 64
Solution:
64 = 2×2×2×2×2×2
By grouping the factors in triplets of equal factors, 64 = (2×2×2)×(2×2×2)
Here, 64 can be grouped into triplets of equal factors.
∴ 64 = 2×2 = 4
Hence, 4 is the cube root of 64.
(ii) 512
Solution:
512 = 2×2×2×2×2×2×2×2×2
By grouping the factors in triplets of equal factors, 512 = (2×2×2)×(2×2×2)×(2×2×2)
Here, 512 can be grouped into triplets of equal factors.
∴ 512 = 2×2×2 = 8
Hence, 8 is the cube root of 512.
(iii) 10648
Solution:
10648 = 2×2×2×11×11×11
By grouping the factors in triplets of equal factors, 10648 = (2×2×2)×(11×11×11)
Here, 10648 can be grouped into triplets of equal factors.
∴ 10648 = 2 ×11 = 22
Hence, 22 is the cube root of 10648.
(iv) 27000
Solution:
27000 = 2×2×2×3×3×3×3×5×5×5
By grouping the factors in triplets of equal factors, 27000 = (2×2×2)×(3×3×3)×(5×5×5)
Here, 27000 can be grouped into triplets of equal factors.
∴ 27000 = (2×3×5) = 30
Hence, 30 is the cube root of 27000.
(v) 15625
Solution:
15625 = 5×5×5×5×5×5
By grouping the factors in triplets of equal factors, 15625 = (5×5×5)×(5×5×5)
Here, 15625 can be grouped into triplets of equal factors.
∴ 15625 = (5×5) = 25
Hence, 25 is the cube root of 15625.
(vi) 13824
Solution:
13824 = 2×2×2×2×2×2×2×2×2×3×3×3
By grouping the factors in triplets of equal factors,
13824 = (2×2×2)×(2×2×2)×(2×2×2)×(3×3×3)
Here, 13824 can be grouped into triplets of equal factors.
∴ 13824 = (2×2× 2×3) = 24
Hence, 24 is the cube root of 13824.
(vii) 110592
Solution:
110592 = 2×2×2×2×2×2×2×2×2×2×2×2×3×3×3
By grouping the factors in triplets of equal factors,
110592 = (2×2×2)×(2×2×2)×(2×2×2)×(2×2×2)×(3×3×3)
Here, 110592 can be grouped into triplets of equal factors.
∴ 110592 = (2×2×2×2 × 3) = 48
Hence, 48 is the cube root of 110592.
(viii) 46656
Solution:
46656 = 2×2×2×2×2×2×3×3×3×3×3×3
By grouping the factors in triplets of equal factors,
46656 = (2×2×2)×(2×2×2)×(3×3×3)×(3×3×3)
Here, 46656 can be grouped into triplets of equal factors.
∴ 46656 = (2×2×3×3) = 36
Hence, 36 is the cube root of 46656.
(ix) 175616
Solution:
175616 = 2×2×2×2×2×2×2×2×2×7×7×7
By grouping the factors in triplets of equal factors,
175616 = (2×2×2)×(2×2×2)×(2×2×2)×(7×7×7)
Here, 175616 can be grouped into triplets of equal factors.
∴ 175616 = (2×2×2×7) = 56
Hence, 56 is the cube root of 175616.
(x) 91125
Solution:
91125 = 3×3×3×3×3×3×3×5×5×5
By grouping the factors in triplets of equal factors, 91125 = (3×3×3)×(3×3×3)×(5×5×5)
Here, 91125 can be grouped into triplets of equal factors.
∴ 91125 = (3×3×5) = 45
Hence, 45 is the cube root of 91125.
2. State true or false.
(i) Cube of any odd number is even.
Solution:
False
(ii) A perfect cube does not end with two zeros.
Solution:
True
(iii) If the cube of a number ends with 5, then its cube ends with 25.
Solution:
False
(iv) There is no perfect cube which ends with 8.
Solution:
False
(v) The cube of a two-digit number may be a three-digit number.
Solution:
False
(vi) The cube of a two-digit number may have seven or more digits.
Solution:
False
(vii) The cube of a single-digit number may be a single-digit number.
Solution:
True
3. You are told that 1,331 is a perfect cube. Can you guess without factorisation what its cube root is? Similarly, guess the cube roots of 4913, 12167, and 32768.
Solution:
(i) By grouping the digits, we get 1 and 331
We know that since the unit digit of the cube is 1, the unit digit of the cube root is 1.
∴ We get 1 as the unit digit of the cube root of 1331.
The cube of 1 matches the number of the second group.
∴ The ten’s digit of our cube root is taken as the unit place of the smallest number.
We know that the unit’s digit of the cube of a number having digit as unit’s place 1 is 1.
∴ ∛1331 = 11
(ii) By grouping the digits, we get 4 and 913
We know that since the unit digit of the cube is 3, the unit digit of the cube root is 7.
∴ we get 7 as the unit digit of the cube root of 4913. We know 13 = 1 and 23 = 8 , 1 >4 >8
Thus, 1 is taken as the tens digit of the cube root.
∴ ∛4913 = 17
(iii) By grouping the digits, we get 12 and 167.
We know that since the unit digit of the cube is 7, the unit digit of the cube root is 3.
∴ 3 is the unit digit of the cube root of 12167 We know 23 = 8 and 33 = 27 , 8 >12 >27
Thus, 2 is taken as the tens digit of the cube root.
∴ ∛12167= 23
(iv) By grouping the digits, we get 32 and 768.
We know that since the unit digit of the cube is 8, the unit digit of the cube root is 2.
∴ 2 is the unit digit of the cube root of 32768. We know 33 = 27 and 43 = 64 , 27 >32 >64
Thus, 3 is taken as the tens digit of the cube root.
∴ ∛32768= 32
The seventh chapter of Class 8 Maths helps the students in finding the cubes and cube roots of different numbers, understanding the difference between cubes and cube roots, and also to have fun with some interesting patterns. The chapter also lets the students understand the process of finding out the cubes and cube roots of a number using the prime factorisation method. Moreover, the chapter also explains the method of finding the cube root of a cube number. Learning the chapter “Cubes and Cube Roots” enables the students to: